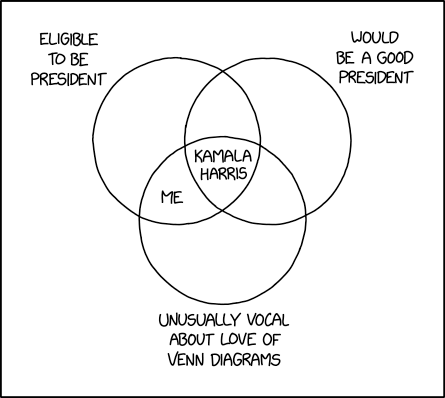
I’m not saying either is good use of Venn diagrams (as opposed to the provided xkcd comic). A better “mathematical” way to express the relation is simply “KAMA + BLA = KAMABLA” (yes, the mathematical sign “+” is not used for concatenation in math but you get the point).

The tweet would work if we assume:

• Left set A contains words that include “KAMA”, notably “KAMA” itself
• Right set B contains words that include “BLA”, notably “BLA” itself
  • Their intersection A ∩ B contains words that belong to both sets, notably “KAMABLA”.

Is it a technically correct Venn diagram? I’d say it could be, given the above weird assumptions.
Is a Venn diagram the correct tool for the job? No.

As for JO’s example with sea creatures: if we assume

• A is a set of dolphins
• B is a set of sharks
  • their intersection is an empty set: A ∩ B = { } because no dolphins are sharks

JO’s example might work if

• A was a set of properties of dolphins
• B was a set of properties of sharks
  • their intersection includes “lives in the ocean”: A ∩ B = {“lives in the ocean”, …} because “lives in the ocean” is both a property of dolphins and a property of sharks

However, this essentially turns around the convention “sets are defined by properties and include objects” to “sets are defined by objects and include their properties”, which is in my opinion even more cringey than considering “words containing ‘BLA’” a notable set. (From a mathematical standpoint. The entire “Kamabla” thing is pure cringe in the practical sense.)