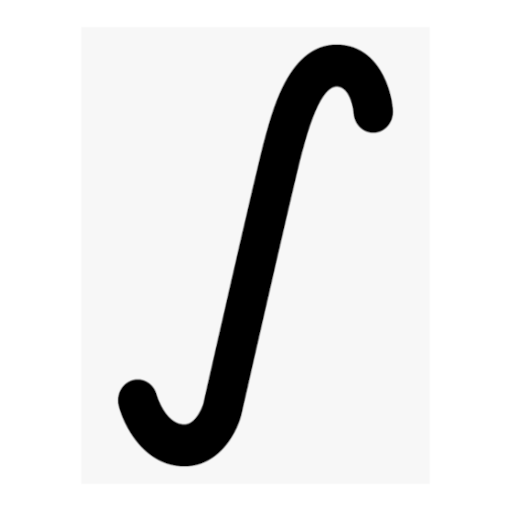
• Show that the infinite multiplication (1+1/1)(1+1/2)(1+1/3)... does not converge.
  • zkfcfbzr@lemmy.worldEnglish
    0·
    6 months ago
    solution

    The terms can be rewritten as:

    (2/1) * (3/2) * (4/3) * … * ((n+1)/n) * …

    Each numerator will cancel with the next denominator. In total everything cancels, so the answer is the empty product, 1.

    …Wait…

    Uhm, ignore that. Rather, consider the products we get when multiplying. We get: 2/1. 6/2. 24/6. Etc. That is, we have:

    Π (n = 1 to k) (n+1)/n = (k+1)! / k! = (k+1)k!/k! = k+1

    k+1 clearly goes to infinity as k → ∞, so our product diverges to infinity.

    • Successful_Try543@feddit.de
      0·
      6 months ago
      solution

      Isn’t this already the result of your 1st formula? As the denominator of the last fraction you wrote down, (n+1)/n, cancels out with the counter of the one right before, n/(n-1), which you didn’t write down. Thus the whole product up to the nth term reads after cancellation of neighbouring counters and denominator pairs (n+1)/1 →∞ when n→∞.